[75]
New lower bounds for the (near) critical Ising and φ4 models' two-point functions. with R. Panis,
preprint.
×
New lower bounds for the (near) critical Ising and φ4 models' two-point functions.
with R. Panis,
preprint.
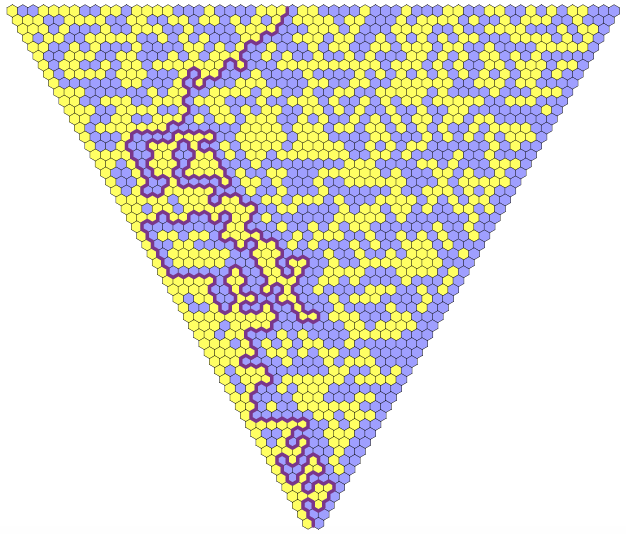
Abstract. We study the nearest-neighbour Ising and φ4 models on ℤd with d≥3 and obtain new lower bounds on their two-point functions at (and near) criticality. Together with the classical infrared bound, these bounds turn into up-to constant estimates when d≥5. When d=4, we obtain an ''almost'' sharp lower bound corrected by a logarithmic factor. As a consequence of these results, we show that η=0 and ν=1/2 when d≥4, where η is the critical exponent associated with the decay of the model's two-point function at criticality and ν is the critical exponent of the correlation length ξ(β). When d=3, we improve previous results and obtain that η≤1/2. As a byproduct of our proofs, we also derive the blow-up at criticality of the so-called bubble diagram when d=3,4.
[75]
An alternative approach for the mean-field behaviour of weakly self-avoiding walks in dimensions d>4. with R. Panis,
preprint.
×
An alternative approach for the mean-field behaviour of weakly self-avoiding walks in dimensions d>4.
with R. Panis,
preprint.
Abstract. This article proposes a new way of deriving mean-field exponents for the weakly self-avoiding walk model in dimensions d>4. Among other results, we obtain up-to-constant estimates for the full-space and half-space two-point functions in the critical and near-critical regimes. A companion paper proposes a similar analysis for spread-out Bernoulli percolation in dimensions d>6.
[74]
An alternative approach for the mean-field behaviour of spread-out Bernoulli percolation in dimensions d>6. with R. Panis,
preprint.
×
An alternative approach for the mean-field behaviour of spread-out Bernoulli percolation in dimensions d>6.
with R. Panis,
preprint.
Abstract. This article proposes a new way of deriving mean-field exponents for sufficiently spread-out Bernoulli percolation in dimensions d>6. We obtain an upper bound for the full-space and half-space two-point functions in the critical and near-critical regimes. In a companion paper, we apply a similar analysis to the study of the weakly self-avoiding walk model in dimensions d>4.
[73]
A characterization of strong percolation via disconnection. with S. Goswami, P.-F. Rodriguez, F. Severo, A. Teixeira,
preprint.
×
A characterization of strong percolation via disconnection.
with S. Goswami, P.-F. Rodriguez, F. Severo, A. Teixeira,
preprint.
Abstract. We consider a percolation model, the vacant set $\mathcal{V}^u$ of random interlacements on $\mathbb Z^d$, $d \geq 3$, in the regime of parameters $u>0$ in which it is strongly percolative. By definition, such values of $u$ pinpoint a robust subset of the super-critical phase, with strong quantitative controls on large local clusters. In the present work, we give a new charaterization of this regime in terms of a single property, monotone in $u$, involving a disconnection estimate for $\mathcal{V}^u$. A key aspect is to exhibit a gluing property for large local clusters from this information alone, and a major challenge in this undertaking is the fact that the conditional law of $\mathcal{V}^u$ exhibits degeneracies. As one of the main novelties of this work, the gluing technique we develop to merge large clusters accounts for such effects. In particular, our methods do not rely on the widely assumed finite-energy property, which the set $\mathcal{V}^u$ does not possess. The charaterization we derive plays a decisive role in the proof of a lasting conjecture regarding the coincidence of various critical parameters naturally associated to $\mathcal{V}^u$ in the companion article [71,72].
[72]
Phase transition for the vacant set of random walk and random interlacements. with S. Goswami, P.-F. Rodriguez, F. Severo, A. Teixeira,
preprint.
×
Phase transition for the vacant set of random walk and random interlacements.
with S. Goswami, P.-F. Rodriguez, F. Severo, A. Teixeira,
preprint.
Abstract. We consider the set of points visited by the random walk on the discrete torus $(\mathbb{Z}/N\mathbb{Z})^d$, for $d \geq 3$, at times of order $uN^d$, for a parameter $u>0$ in the large-$N$ limit. We prove that the vacant set left by the walk undergoes a phase transition across a non-degenerate critical value $u_* = u_*(d)$, as follows. For all $u< u_*$, the vacant set contains a giant connected component with high probability, which has a non-vanishing asymptotic density and satisfies a certain local uniqueness property. In stark contrast, for all $u> u_*$ the vacant set scatters into tiny connected components. Our results further imply that the threshold $u_*$ precisely equals the critical value, introduced by Sznitman in \textit{Ann.~Math., 171 (2010), 2039--2087}, which characterizes the percolation transition of the corresponding local limit, the vacant set of random interlacements on $\mathbb Z^d$. Our findings also yield the analogous infinite-volume result, i.e.~the long purported equality of three critical parameters $\bar u$, $u_*$ and $u_{**}$ naturally associated to the vacant set of random interlacements.
[71]
Finite range interlacements and couplings. with S. Goswami, P.-F. Rodriguez, F. Severo, A. Teixeira,
preprint.
×
Finite range interlacements and couplings.
with S. Goswami, P.-F. Rodriguez, F. Severo, A. Teixeira,
preprint.
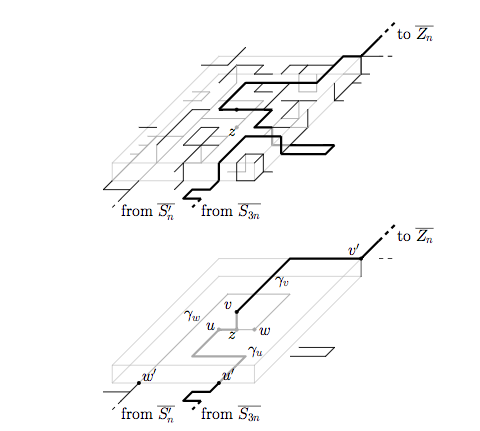
Abstract. In this article, we consider the interlacement set $\mathcal{I}^u$ at level $u>0$ on $\mathbb Z^d$, $d \geq3$, and its finite range version $\mathcal{I}^{u,L}$ for $L >0$, given by the union of the ranges of a Poisson cloud of random walks on $\mathbb Z^d$ having intensity $u/L$ and killed after $L$ steps.
As $L\to \infty$, the random set $\mathcal{I}^{u,L}$ has a non-trivial (local) limit, which is precisely $\mathcal{I}^u$.
A natural question is to understand how the sets $\mathcal{I}^{u,L}$ and $\mathcal{I}^{{u}}$ can be related, if at all,~in such a way that their intersections with a box of large radius $R$ almost coincide. We address this question, which depends sensitively on $R$, by developing couplings allowing for a similar comparison to hold with very high probability for $\mathcal{I}^{u,L}$ and $\mathcal{I}^{{u'},2L}$, with $u' \approx u$. In particular, for the vacant set $\mathcal{V}^u=\mathbb Z^d \setminus \mathcal{I}^u$ with values of $u$ near the critical threshold, our couplings remain effective at scales $R \gg \sqrt{L}$, which corresponds to a natural barrier across which the walks of length $L$ comprised in $\mathcal{I}^{u,L}$ \textit{de-solidify} inside $B_R$, i.e.~lose their intrinsic long-range structure to become increasingly `dust-like'. These mechanisms are complementary to the \textit{solidification} effects recently exhibited. By iterating the resulting couplings over dyadic scales $L$, the models $\mathcal{I}^{u,L}$ are seen to constitute a stationary finite range approximation of $\mathcal{I}^u$ at large spatial scales near the critical point $u_*$.
Among others, these couplings are important ingredients for the characterization of the phase transition for percolation of the vacant sets of random walk and random interlacements in the companion articles [72,73].
[70]
Sharp metastability transition for two-dimensional bootstrap percolation with symmetric isotropic threshold rules. with I.
Hartarsky,
PTRF.
×
Sharp metastability transition for two-dimensional bootstrap percolation with symmetric isotropic threshold rules.
with I. Hartarsky
Probability Theory and Related Fields
, 190, 445–483, 2024.
Abstract. We study two-dimensional critical bootstrap percolation models. We establish that a class of these models including all isotropic threshold rules with a convex symmetric neighbourhood, undergoes a sharp metastability transition. This extends previous instances proved for several specific rules. The paper supersedes a draft by Alexander Holroyd and the first author from 2012. While it served a role in the subsequent development of bootstrap percolation universality, we have chosen to adopt a more contemporary viewpoint in its present form.
[69]
Near
critical scaling relations for planar Bernoulli
percolation without differential inequalities. with I.
Manolescu and V. Tassion,
preprint.
×
Near critical scaling relations for planar Bernoulli
percolation without differential inequalities.
with I. Manolescu and V. Tassion
preprint.
Abstract. We provide a new proof of the
near-critical scaling relation $\beta=\xi_1\nu$ for
Bernoulli percolation on the square lattice already
proved by Kesten in 1987. We rely on a novel approach
that does not invoke Russo's formula, but rather relates
differences in crossing probabilities at different
scales. The argument is shorter and more robust than
previous ones and is more likely to be adapted to other
models. The same approach may be used to prove the other
scaling relations appearing in Kesten's work.
[68]
Existence of an
unbounded nodal hypersurface for smooth Gaussian fields in
dimension $d\ge 3$. with A. Rivera, P.F. Rodriguez, and H.
Vanneuville,
Ann. Probab.
×
Existence of an unbounded nodal hypersurface for smooth
Gaussian fields in dimension $d\ge 3$.
with A. Rivera, P.F. Rodriguez, and H. Vanneuville
Annals of Probability
, 51(1), 228-276, 2023.
Abstract. For the Bargmann--Fock field on
$\mathbb R^d$ with $d\ge3$, we prove that the critical
level $\ell_c(d)$ of the percolation model formed by the
excursion sets $\{ f \ge \ell \}$ is strictly positive.
This implies that for every $\ell$ sufficiently close to
0 (in particular for the nodal hypersurfaces
corresponding to the case $\ell=0$), $\{f=\ell\}$
contains an unbounded connected component that visits
``most'' of the ambient space. Our findings actually
hold for a more general class of positively correlated
smooth Gaussian fields with rapid decay of correlations.
The results of this paper show that the behaviour of
nodal hypersurfaces of these Gaussian fields in $\mathbb
R^d$ for $d\ge3$ is very different from the behaviour of
nodal lines of their two-dimensional analogues.
[67]
Conformal
invariance of double random currents I: identification of the
limit with M. Lis and W. Qian,
preprint.
×
Conformal invariance of double random currents I:
identification of the limit.
with M. Lis and W. Qian,
preprint.
Abstract. This is the first of two papers devoted
to the proof of conformal invariance of the critical
double random current model on the square lattice. More
precisely, we show the convergence of loop ensembles
obtained by taking the cluster boundaries in the sum of
two independent currents with free and wired boundary
conditions. The strategy is first to prove convergence
of the associated height function to the continuum
Gaussian free field, and then to characterize the
scaling limit of the loop ensembles as certain local
sets of this Gaussian Free Field. In this paper, we
identify uniquely the possible subsequential limits of
the loop ensembles. Combined with the second paper, this
completes the proof of conformal invariance.
[66]
Conformal
invariance of double random currents II: tightness and
properties in the discrete with M. Lis and W. Qian,
preprint.
×
Conformal invariance of double random currents II:
tightness and properties in the discrete.
with M. Lis and W. Qian,
preprint.
Abstract. This is the second of two papers
devoted to the proof of conformal invariance of the
critical double random current on the square lattice.
More precisely, we show convergence of loop ensembles
obtained by taking the cluster boundaries in the sum of
two independent critical currents (both for free and
wired boundary conditions). The strategy is first to
prove convergence of the associated height function to
the continuum Gaussian free field, and then to
characterize the scaling limit of the loop ensembles as
certain local sets of this Gaussian Free Field. In this
paper, we derive crossing properties of the discrete
model required to prove this characterization.
[65]
Rotational
invariance in critical planar lattice models with K.K.
Kozlowski, D. Krachun, I. Manolescu, and M. Oulamara,
preprint.
×
Rotational invariance in critical planar lattice models.
with K.K. Kozlowski, D. Krachun, I. Manolescu, and M.
Oulamara,
preprint.
Abstract. In this paper, we prove that the large
scale properties of a number of two-dimensional lattice
models are rotationally invariant. More precisely, we
prove that the random-cluster model on the square
lattice with cluster-weight $1\le q\le 4$ exhibits
rotational invariance at large scales. This covers the
case of Bernoulli percolation on the square lattice as
an important example. We deduce from this result that
the correlations of the Potts models with
$q\in\{2,3,4\}$ colors and of the six-vertex height
function with $\Delta\in[-1,-1/2]$ are rotationally
invariant at large scales.
[64]
Delocalization of
the height function of the six-vertex model with A.
Karrila, I. Manolescu, and M. Oulamara,
JEMS.
×
Delocalization of the height function of the six-vertex
model.
with A. Karrila, I. Manolescu, and M. Oulamara,
Journal of the EMS
, 26(11), 1-60, 2024.
Abstract. We show that the height function of the
six-vertex model, in the parameter range $\mathbf
a=\mathbf b=1$ and $\mathbf c\ge1$, is delocalized with
logarithmic variance when $\mathbf c\le 2$. This
complements the earlier proven localization for $\mathbf
c>2$. Our proof relies on Russo--Seymour--Welsh type
arguments, and on the local behaviour of the free energy
of the cylindrical six-vertex model, as a function of
the unbalance between the number of up and down arrows.
[63]
On the six-vertex
model’s free energy with K.K. Kozlowski, D. Krachun, I.
Manolescu, and T. Tikhonovskaia,
CIMP.
×
On the six-vertex model’s free energy.
with K.K. Kozlowski, D. Krachun, I. Manolescu, and T.
Tikhonovskaia,
Communications in Mathematical Physics
, 395, 1383–1430, 2022.
Abstract. In this paper, we provide new proofs of
the existence and the condensation of Bethe roots for
the Bethe Ansatz equation associated with the six-vertex
model with periodic boundary conditions and an arbitrary
density of up arrows (per line) in the regime
$\Delta<1$. As an application, we provide a short,
fully rigorous computation of the free energy of the
six-vertex model on the torus, as well as an asymptotic
expansion of the six-vertex partition functions when the
density of up arrows approaches $1/2$. This latter
result is at the base of a number of recent results, in
particular the rigorous proof of
continuity/discontinuity of the phase transition of the
random-cluster model, the localization/delocalization
behaviour of the six-vertex height function when $a=b=1$
and $c\ge1$, and the rotational invariance of the
six-vertex model and the Fortuin-Kasteleyn percolation.
[62]
Planar
random-cluster model: scaling relations with I. Manolescu,
Forum of Mathematics, Pi.
×
Planar random-cluster model: scaling relations,
Forum of Mathematics, Pi, Volume 10 , 2022 , e23 (85 pages).
with I. Manolescu,
preprint.
Abstract. This paper studies the critical and
near-critical regimes of the planar random-cluster model
on $\mathbb Z^2$ with cluster-weight$q\in[1,4]$ using
novel coupling techniques. More precisely, we derive the
scaling relations between the critical exponents
$\beta$, $\gamma$, $\delta$, $\eta$, $\nu$, $zeta$ as
well as $\alpha$ (when $\alpha\ge0$). As a key input, we
show the stability of crossing probabilities in the
near-critical regime using new interpretations of the
notion of influence of an edge in terms of the rate of
mixing. As a byproduct, we derive a generalization of
Kesten's classical scaling relation for Bernoulli
percolation involving the ``mixing rate'' critical
exponent $\iota$ replacing the four-arm event exponent
$\xi_4$.
[61]
Long-range order
for critical Book-Ising and Book-percolation with C.
Garban and V. Tassion,
CIMP.
×
Long-range order for critical Book-Ising and
Book-percolation,
with C. Garban and V. Tassion,
Communications in Mathematical Physics.
, 404, 1309–1339, 2023.
Abstract. In this paper, we investigate the
behaviour of statistical physics models on a book with
pages that are isomorphic to half-planes. We show that
even for models undergoing a continuous phase transition
on $\mathbb Z^2$, the phase transition becomes
discontinuous as soon as the number of pages is
sufficiently large. In particular, we prove that the
Ising model on a three pages book has a discontinuous
phase transition (if one allows oneself to consider
large coupling constants along the line on which pages
are glued). Our work confirms predictions in theoretical
physics which relied on renormalization group, conformal
field theory and numerics ([Car91,ITB91,SMP10]) some of
which were motivated by the analysis of the Renyi
entropy of certain quantum spin systems.
[60]
Long-range models
in 1D revisited with C. Garban and V. Tassion,
preprint.
×
Long-range models in 1D revisited.
with C. Garban and V. Tassion,
preprint.
Abstract. In this short note, we revisit a number
of classical results on long-range 1D percolation, Ising
model and Potts models [FS82, NS86, ACCN88, IN88]. More
precisely, we show that for Bernoulli percolation, FK
percolation and Potts models, there is symmetry breaking
for the $1/r^2$-interaction at large $\beta$, and that
the phase transition is necessarily discontinuous. We
also show, following the notation of [ACCN88] that
$\beta^*(q)=1$ for all $q\ge1$.
[59]
Logarithmic
variance for the height function of square-ice with M.
Harel, B. Laslier, A. Raoufi, G. Ray,
CIMP.
×
Logarithmic variance for the height function of
square-ice.
with M. Harel, B. Laslier, A. Raoufi, G. Ray,
Communications in Mathematical Physics,
396, 867–902, 2022.
Abstract. In this article, we prove that the
height function associated with the square-ice model
(i.e.~the six-vertex model with $a=b=c=1$ on the square
lattice), or, equivalently, of the uniform random
homomorphisms from $\mathbb Z^2$ to $\mathbb Z$, has
logarithmic variance. This establishes a strong form of
roughness of this height function.
[58]
Equality of
critical parameters for percolation of Gaussian free field
level-sets with S. Goswami, P.F. Rodriguez and F. Severo,
Duke Mathematical Journal.
×
Equality of critical parameters for percolation of
Gaussian free field level-sets.
with S. Goswami, P.F. Rodriguez and F. Severo,
Duke
Mathematical Journal, 172(5): 839-913, 2023.
Abstract. We consider level-sets of the Gaussian
free field on $\mathbb Z^d$, for $d\geq 3$, above a
given real-valued height parameter $h$. As $h$ varies,
this defines a canonical percolation model with strong,
algebraically decaying correlations. We prove that three
natural critical parameters associated to this model,
namely $h_{**}(d)$, $h_{*}(d)$ and $\bar h(d)$,
respectively describing a well-ordered subcritical
phase, the emergence of an infinite cluster, and the
onset of a local uniqueness regime in the supercritical
phase, actually coincide, i.e. $h_{**}(d)=h_{*}(d)= \bar
h(d)$ for any $d \geq 3$. At the core of our proof lies
a new interpolation scheme aimed at integrating out the
long-range dependence of the Gaussian free field. The
successful implementation of this strategy relies
extensively on certain novel renormalization techniques,
in particular to control so-called large-field effects.
This approach opens the way to a complete understanding
of the off-critical phases of strongly correlated
percolation models.
[57]
Existence of phase
transition for percolation using the Gaussian Free Field
with S. Goswami, A. Raoufi, F. Severo and A. Yadin,
Duke
Mathematical Journal.
×
Existence of phase transition for percolation using the
Gaussian Free Field.
with Subhajit Goswami, Aran Raoufi, Franco Severo, Ariel
Yadin,
Duke Mathematical
Journal 169(18), 3539-3563, 2020.
Abstract. In this paper, we prove that Bernoulli
percolation on bounded degree graphs with isoperimetric
dimension $d>4$ undergoes a non-trivial phase
transition (in the sense that $p_c<1$). As a
corollary, we obtain that the critical point of
Bernoulli percolation on infinite quasi-transitive
graphs (in particular, Cayley graphs) with super-linear
growth is strictly smaller than 1, thus answering a
conjecture of Benjamini and Schramm. The proof relies on
a new technique consisting in expressing certain
functionals of the Gaussian Free Field (GFF) in terms of
connectivity probabilities for percolation model in a
random environment. Then, we integrate out the
randomness in the edge-parameters using a multi-scale
decomposition of the GFF. We believe that a similar
strategy could lead to proofs of the existence of a
phase transition for various other models.
[56]
Upper bounds on
the percolation correlation length with G.Kozma and V.
Tassion,
special volume in memory of Vladas Sidoravicius
published in Birkhauser series "Progress in Probability".
×
Upper bounds on the percolation correlation length.
with G. Kozma and V. Tassion,
special
volume in memory of Vladas Sidoravicius published in
Birkhauser series "Progress in Probability"Out of Equilibrium 3: Celebrating Vladas Sidoravicius. Progress in Probability, vol 77.
Abstract. We study the size of the near-critical
window for Bernoulli percolation on $\mathbb Z^d$. More
precisely, we use a quantitative Grimmett-Marstrand
theorem to prove that the correlation length, both below
and above criticality, is bounded from above by
$\exp(C/|p-p_c|^2)$. Improving on this bound would be a
further step towards the conjecture that there is no
infinite cluster at criticality on $\mathbb Z^d$ for
every $d\ge2$.
[55]
Universality of
two-dimensional critical cellular automata with B.
Bollobás, R. Morris and P. Smith
PLMS.
×
Universality of two-dimensional critical cellular
automata.
with B. Bollobás, R. Morris and P. Smith,
Proceedings of the London Mathematical Society
, 126(2), 620-793, 2023.

Abstract. We study the class
of monotone, two-state, deterministic cellular
automata, in which sites are activated (or `infected')
by certain con�gurations of nearby infected sites.
These models have close connections to statistical
physics, and several specifi�c examples have been
extensively studied in recent years by both
mathematicians and physicists. This general setting
was �first studied only recently, however, by
Bollob�as, Smith and Uzzell, who showed that the
family of all such `bootstrap percolation' models on
$\mathbb Z^2$ can be naturally partitioned into three
classes, which they termed subcritical, critical and
supercritical.
In this paper we determine the order of the threshold
for percolation (complete occupation) for every
critical bootstrap percolation model in two
dimensions. This `universality' theorem includes as
special cases results of Aizenman and Lebowitz,
Gravner and Gri�eath, Mountford, and van Enter and
Hulshof, signi�cantly strengthens bounds of Bollob�as,
Smith and Uzzell, and complements recent work of
Balister, Bollob�as, Przykucki and Smith on
subcritical models.
[54]
Discontinuity of
the phase transition for the planar random-cluster and Potts
models with $q>4$ with M. Gagnebin, M. Harel, I.
Manolescu and V. Tassion.
Annales de l'ENS.
×
Discontinuity of the phase transition for the planar
random-cluster and Potts models with $q>4$.
with Maxime Gagnebin, Matan Harel, Ioan Manolescu and
Vincent Tassion,
Annales
de l'ENS, 54(6), 1363-1413, 2021.

© Vincent Beffara
Abstract. We prove that the
$q$-state Potts model and the random-cluster model
with cluster weight $q>4$ undergo a discontinuous
phase transition on the square lattice. More
precisely, we show
1. Existence of multiple infinite-volume measures for
the critical Potts and random-cluster models,
2. Ordering for the measures with monochromatic (resp.
wired) boundary conditions for the critical Potts
model (resp. random-cluster model), and
3. Exponential decay of correlations for the measure
with free boundary conditions for both the critical
Potts and random-cluster models. The proof is based on
a rigorous computation of the Perron-Frobenius
eigenvalues of the diagonal blocks of the transfer
matrix of the six-vertex model, whose ratios are then
related to the correlation length of the
random-cluster model. As a byproduct, we rigorously
compute the correlation lengths of the critical
random-cluster and Potts models, and show that they
behave as $\exp(\pi^2/\sqrt{q-4})$ as~$q$ tends to 4.
[53]
Renormalization of
crossing probabilities in the planar random-cluster model
with V. Tassion.
Dobrushin's issue of Moscow Math. journal.
×
Renormalization of crossing probabilities in the planar
random-cluster model.
with V. Tassion,
Dobrushin's
issue of Moscow Math. journal, 24 January 2019 .
Abstract. The study of crossing probabilities -
i.e. probabilities of existence of paths crossing
rectangles - has been at the heart of the theory of
two-dimensional percolation since its beginning. They
may be used to prove a number of results on the model,
including speed of mixing, tails of decay of the
connectivity probabilities, scaling relations, etc. In
this article, we develop a renormalization scheme for
crossing probabilities in the two-dimensional
random-cluster model. The outcome of the process is a
precise description of an alternative between four
behaviors:
- Subcritical: Crossing probabilities, even with
favorable boundary conditions, converge exponentially
fast to 0.
- Supercritical: Crossing probabilities, even with
unfavorable boundary conditions, converge exponentially
fast to 1.
- Critical discontinuous: Crossing probabilities
converge to 0 exponentially fast with unfavorable
boundary conditions and to 1 with favorable boundary
conditions.
- Critical continuous: Crossing probabilities remain
bounded away from 0 and 1 uniformly in the boundary
conditions.
The novelty of the approach is that the proof does not
rely on self-duality, enabling it to apply in a much
larger generality, including the random-cluster model on
arbitrary graphs with sufficient symmetry, but also
other models than the random-cluster model such as some
random height models.
[52]
Planar
random-cluster model: fractal properties of the critical phase
with I. Manolescu and V. Tassion.
PTRF.
×
Planar random-cluster model: fractal properties of the
critical phase,
Probability Theory and Related
Fields, 181, 301--339, 2021.
with I. Manolescu and V. Tassion,
Probability
Theory and Related Fields (to appear)
Abstract. This paper is studying the critical
regime of the planar random-cluster model on $\mathbb
Z^2$ with cluster-weight $q\in[1,4)$. More precisely, we
prove crossing estimates in quads which are uniform in
their boundary conditions and depend only on their
extremal lengths. They imply in particular that any
fractal boundary is touched by macroscopic clusters,
uniformly in its roughness or the configuration on said
boundary. Additionally, they imply that any
sub-sequential scaling limit of the collection of
interfaces between primal and dual clusters is made of
loops that are non-simple. We also obtain a number of
properties of so-called arm-events: three universal
critical exponents (two arms in the half-plane, three
arms in the half-plane and five arms in the bulk),
quasi-multiplicativity and well-separation properties
(even when arms are not alternating between primal and
dual), and the fact that the four-arm exponent is
strictly smaller than 2. These results were previously
known only for Bernoulli percolation ($q=1$) and the
FK-Ising model ($q=2$). Finally, we prove new bounds on
the one, two and four arms exponents for $q\in[1,2]$.
These improve the previously known bounds, even for
Bernoulli percolation.
[51]
Marginal
triviality of the scaling limits of critical 4D Ising and
$\varphi_4^4$ models with M. Aizenman.
Ann. Math.
×
Marginal triviality of the scaling limits of critical 4D
Ising and $\varphi_4^4$ models.
with M. Aizenman,
Annals
of Mathematics , 194(1), 163-235, 2021.
Abstract. We prove that the scaling limits of
spin fluctuations in four-dimensional Ising-type models
with nearest-neighbor ferromagnetic interaction at or
near the critical point are Gaussian. A similar
statement is proven for the $\lambda \phi^4$ fields over
$\mathbb{R}^4$ with a lattice ultraviolet cutoff, in the
limit of infinite volume and vanishing lattice spacing.
The proofs are enabled by the models' random current
representation, in which the correlation functions'
deviation from Wick's law is expressed in terms of
intersection probabilities of random currents with
sources at distances which are large on the model's
lattice scale. Guided by the analogy with random walk
intersection amplitudes, the analysis focuses on the
improvement of the so-called tree diagram bound by a
logarithmic correction term, which is derived here
through multi-scale analysis.
[50]
Macroscopic loops
in the loop $O(n)$ model at Nienhuis' critical point with
A. Glazman, R. Peled and Y. Spinka
JEMS.
×
Macroscopic loops in the loop $O(n)$ model at Nienhuis'
critical point.
with Alexander Glazman, Ron Peled and Yinon Spinka,
Journal
of European Mathematical Society, 23(1). 315-347,
2020.
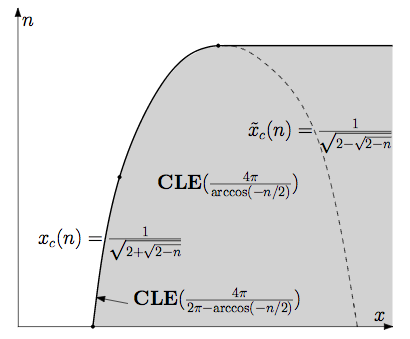
Abstract. The loop $O(n)$
model is a model for a random collection of
non-intersecting loops on the hexagonal lattice, which
is believed to be in the same universality class as
the spin $O(n)$ model. It has been predicted by
Nienhuis that for $0\le n\le 2$ the loop $O(n)$ model
exhibits a phase transition at a critical parameter
$x_c(n)=1/\sqrt{2+\sqrt{2-n}}$. For $0 < n\le 2$,
the transition line has been further conjectured to
separate a regime with short loops when $x <
x_c(n)$ from a regime with macroscopic loops when
$x\ge x_c(n)$. In this paper, we prove that for $n\in
[1,2]$ and $x=x_c(n)$ the loop $O(n)$ model exhibits
macroscopic loops. This is the first instance in which
a loop $O(n)$ model with $n\neq 1$ is shown to exhibit
such behaviour. A main tool in the proof is a new
positive association (FKG) property shown to hold when
$n \ge 1$ and $0 < x\le 1/\sqrt{n}$. This property
implies, using techniques recently developed for the
random-cluster model, the following dichotomy: either
long loops are exponentially unlikely or the origin is
surrounded by loops at any scale (box-crossing
property). We develop a ``domain gluing'' technique
which allows us to employ Smirnov's parafermionic
observable to rule out the first alternative when
$x=x_c(n)$ and $n\in[1,2]$.
[49]
A note on
Schramm's locality conjecture for random-cluster models
with V. Tassion
Sojourns in Probability Theory and
Statistical Physics - II.
×
A note on Schramm's locality conjecture for
random-cluster models.
with Vincent Tassion,
Sojourns
in Probability Theory and Statistical Physics - II,
123–134, 2019.
Abstract. In this note, we
discuss a generalization of Schramm's locality
conjecture to the case of random-cluster models. We give
some partial (modest) answers, and present several
related open questions. Our main result is to show that
the critical inverse temperature of the Potts model on
$\mathbb Z^r\times(\mathbb Z/2n\mathbb Z)^{d-r}$ (with
$r\ge3$) converges to the critical inverse temperature
of the model on $\mathbb Z^d$ as $n$ tends to infinity.
Our proof relies on the infrared bound and, contrary to
the equivalent (harder) statement for Bernoulli
percolation, does not involve renormalization arguments.
[48]
Subcritical phase
of $d$-dimensional Poisson-Boolean percolation and its vacant
set with A. Raoufi and V. Tassion
AHL.
×
Subcritical phase of $d$-dimensional Poisson-Boolean
percolation and its vacant set.
with Aran Raoufi, Vincent Tassion,
to
appaer in Annales Henri Lebesgue, 3, 677–700, 2020.
credit: wikipedia
Abstract. We prove that the
Poisson-Boolean percolation on $\mathbb{R}^d$
undergoes a sharp phase transition in any dimension
under the assumption that the radius distribution has
a $5d-3$ finite moment (in particular we do not assume
that the distribution is bounded). More precisely, we
prove that: -In the whole subcritical regime, the
expected size of the cluster of the origin is finite,
and furthermore we obtain bounds for the origin to be
connected to distance $n$: when the radius
distribution has a finite exponential moment, the
probability decays exponentially fast in $n$, and when
the radius distribution has heavy tails, the
probability is equivalent to the probability that the
origin is covered by a ball going to distance $n$. -
In the supercritical regime, it is proved that the
probability of the origin being connected to infinity
satisfies a mean-field lower bound. The same proof
carries on to conclude that the vacant set of
Poisson-Boolean percolation on $\mathbb{R}^d$
undergoes a sharp phase transition. This paper belongs
to a series of papers using the theory of randomized
algorithms to prove sharpness of phase transitions.
[47]
On the number of
maximal paths in directed last-passage percolation with H.
Kesten, F. Nazarov, Y. Peres, V. Sidoravicius
Ann. Probab..
×
On the number of maximal paths in directed last-passage
percolation.
with Harry Kesten, Fedor Nazarov, Yuval Peres, Vladas
Sidoravicius,
Annals of
Probability, 48(5), 2176–2188, 2020.
Abstract. We show that the number of maximal
paths in directed last-passage percolation on the
hypercubic lattice $\mathbb Z^d$ ($d\ge2$) in which
weights take finitely many values is typically
exponentially large.
[46]
Bounding the
number of self-avoiding walks: Hammersley-Welsh with polygon
insertion with S. Ganguly, A. Hammond and I. Manolescu
AOP.
×
Bounding the number of self-avoiding walks:
Hammersley-Welsh with polygon insertion.
with S. Ganguly, A. Hammond and I. Manolescu,
Annals
of Probability, 48(4), 1644–1692, 2020.
Abstract.
Let $c_n = c_n(d)$ denote the
number of self-avoiding walks of length~$n$ starting
at the origin in the Euclidean nearest-neighbour
lattice~$\mathbb Z^d$. Let $\mu = \lim_n c_n^{1/n}$
denote the connective constant of~$\mathbb Z^d$. In
1962, Hammersley and Welsh proved that, for each $d
\geq 2$, there exists a constant $C > 0$ such that
$c_n \leq \exp(C n^{1/2}) \mu^n$ for all $n \in
\mathbb N$. While it is anticipated that $c_n
\mu^{-n}$ has a power-law growth in $n$, the best
known upper bound in dimension two has remained of the
form $n^{1/2}$ inside the exponential. We consider two
planar lattices and prove that $c_n \leq \exp(C n^{1/2
-\epsilon}) \mu^n$ for an explicit constant
$\epsilon> 0$ (where here $\mu$ denotes the
connective constant for the lattice in question). The
result is conditional on a lower bound on the number
of self-avoiding polygons of length~$n$, which is
proved to hold on the hexagonal lattice~$\mathbb H$
for all $n$, and subsequentially in~$n$ for $\mathbb
Z^2$. A power-law upper bound on $c_n \mu^{-n}$ for
$\mathbb H$ is also proved, contingent on a
non-quantitative assertion concerning this lattice's
connective constant.
[45]
Dimerization and
Néel order in different quantum spin chains through a shared
loop representation with M. Aizenman and S. Warzel.
Annales
de l'IHP D.
×
Dimerization and Néel order in different quantum spin
chains through a shared loop representation.
with M. Aizenman and S. Warzel,
Annales
de l'IHP D, 21, 2737–2774, 2020.
Abstract. The ground states of the spin-$S$
antiferromagnetic chain $H_\mathrm{AF}$ with a
projection-based interaction and the spin-$1/2$
XXZ-chain $H_\mathrm{XXZ}$ at anisotropy parameter
$\Delta=\cosh(\lambda)$ share a common loop
representation in terms of a two-dimensional functional
integral which is similar to the classical planar
$Q$-state Potts model at $\sqrt Q= 2S+1
=2\cosh(\lambda)$. The multifaceted relation is used
here to directly relate the distinct forms of
translation symmetry breaking which are manifested in
the ground states of these two models: dimerization for
$H_\mathrm{AF}$ at all $S>1/2$, and N\'eel order for
$H_\mathrm{XXZ}$ at $\lambda >0$. The results
presented include: i) a translation to the above quantum
spin systems of the results which were recently proven
by Duminil-Copin-Li-Manolescu for a broad class of
two-dimensional random-cluster models, and ii) a short
proof of the symmetry breaking in a manner similar to
the recent structural proof by Ray-Spinka of the
discontinuity of the phase transition for $Q>4$.
Altogether, the quantum manifestation of the change
between $Q=4$ and $Q>4$ is a transition from a
gapless ground state to a pair of gapped and extensively
distinct ground states.
[44]
Internal
Diffusion-Limited aggregation with uniform starting points
with I. Benjamini, G. Kozma and C. Lucas,
AIHP.
×
Internal Diffusion-Limited aggregation with uniform
starting points.
with Itai Benjamini, Gady Kozma and Cyrille Lucas,
Annales
de l'Institut Henri Poincaré Prob. Stat. 56(1), 391-404,
2020.
Abstract. We study internal
diffusion-limited aggregation with random starting
points on $\mathbb Z^d$. In this model, each new
particle starts from a vertex chosen uniformly at random
on the existing aggregate. We prove that the limiting
shape of the aggregate is a Euclidean ball.
[43]
Exponential decay
of truncated correlations for the Ising model in any dimension
for all but the critical temperature with S. Goswami and
A. Raoufi,
CIMP.
×
Exponential decay of truncated correlations for the
Ising model in any dimension for all but the critical
temperature.
with Subhajit Goswami, Aran Raoufi,
Communications
in Mathematical Physics, 374(2), 891–921, 2020.
Abstract. The truncated two-point function of the
ferromagnetic Ising model on $\mathbb Z^d$ ($d\ge3$) in
its pure phases is proven to decay exponentially fast
throughout the ordered regime ($\beta>\beta_c$ and
$h=0$). Together with the previously known results, this
implies that the exponential clustering property holds
throughout the model's phase diagram except for the
critical point: $(\beta,h) = (\beta_c,0)$.
[42]
On the double
random current nesting field with M. Lis,
PTRF.
×
On the double random current nesting field.
with Marcin Lis,
Probability
Theory and Related Fields, 175(3-4), 937-955, 2019.
Abstract. We relate the
planar random current representation introduced by
Griffiths, Hurst and Sherman to the dimer model. More
precisely, we provide a measure-preserving map between
double random currents (obtained as the sum of two
independent random currents) on a planar graph and
dimers on an associated bipartite graph. We also
construct a nesting field for the double random
current, which, under this map, corresponds to the
height function of the dimer model. As applications,
we provide an alternative derivation of some of the
bozonization rules obtained recently by Dub\'edat, and
show that the spontaneous magnetization of the Ising
model on a planar biperiodic graph vanishes at
criticality.

[41]
Exponential decay
of connection probabilities for subcritical Voronoi
percolation in $\mathbb R^d$ with A. Raoufi and V.
Tassion,
PTRF.
×
Exponential decay of connection probabilities for
subcritical Voronoi percolation in $\mathbb R^d$.
with Aran Raoufi and Vincent Tassion,
Probability
Theory and Related Fields, 173(1–2), 479–490, 2019.
Abstract. We prove that for
Voronoi percolation on $\mathbb R^d$, there exists
$p_c\in[0,1]$ such that
- for $p$ smaller than $p_c$, there exists $c_p>0$
such that $\mathbb P_p[0\text{ connected to distance
}n]\le \exp(-c_pn)$,
- there exists $c>0$ such that for $p>p_c$,
$\mathbb P_p[0\text{ connected to }\infty]\ge
c(p-p_c)$.
This result offers a new way of showing that
$p_c=1/2$, and the first proof of mean-field lower
bound for the density of the infinite cluster, even in
dimension 2. This paper belongs to a series of papers
using the theory of algorithms to prove sharpness of
the phase transition.
[40]
Minimal growth
harmonic functions on lamplighter groups with I.
Benjamini, G. Kozma and A. Yadin,
New
York J Math.
×
Minimal growth harmonic functions on lamplighter groups.
with I. Benjamini, G. Kozma and A. Yadin,
New
York Journal of Mathematics, 23, 833--858, 2017.
Abstract. We study the minimal
possible growth of harmonic functions on lamplighters. We
find that $(\mathbb Z/2)\wr \mathbb Z$ has no sublinear
harmonic functions, $(\mathbb Z/2)\wr \mathbb Z^2$ has no
sublogarithmic harmonic functions, and neither has the
repeated wreath product $(\dotsb(\mathbb Z/2\wr\mathbb
Z^2)\wr\mathbb Z^2)\wr\dotsb\wr\mathbb Z^2$. These results
have implications on attempts to quantify the
Derriennic-Kaimanovich-Vershik theorem.
[39]
Emergent Planarity
in two-dimensional Ising Models with finite-range Interactions
with M. Aizenman, V. Tassion and S. Warzel,
Inventiones.
×
Emergent Planarity in two-dimensional Ising Models with
finite-range Interactions.
with Michael Aizenman, Vincent Tassion and Simone Warzel,
Inventiones
Mathematicae, 216(3), 661-743, 2019.
Abstract. The known
Pfaffian structure of the boundary spin correlations,
and more generally order-disorder correlation
functions, is given a new explanation through simple
topological considerations within the model's random
current representation. This perspective is then
employed in the proof that the Pfaffian structure of
boundary correlations emerges asymptotically at
criticality in Ising models on $\mathbb Z^2$ with
finite-range interactions. The analysis is enabled by
new results on the stochastic geometry of the
corresponding random currents. The proven statement
establishes an aspect of universality, seen here in
the emergence of fermionic structures in two
dimensions beyond the solvable cases.
[38]
A new computation
of the critical point for the planar random-cluster model with
$q\ge1$ with A. Raoufi and V. Tassion,
Ann.
IHP.
×
A new computation of the critical point for the planar
random-cluster model with $q\ge1$.
with A. Raoufi and V. Tassion,
Annales
de l'IHP, 54(1), 422–436, 2018.
Abstract. We present a new
computation of the critical value of the random-cluster
model with cluster weight $q\ge 1$ on $\mathbb Z^2$. This
provides an alternative approach to the result obtained by
Beffara and Duminil-Copin. We believe that this approach
has several advantages. First, most of the proof can
easily be extended to other planar graphs with sufficient
symmetries. Furthermore, it invokes RSW-type arguments
which are not based on self-duality. And finally, it
contains a new way of applying sharp threshold results
which avoid the use of symmetric events and periodic
boundary conditions. Some of the new methods presented in
this paper have a larger scope than the planar
random-cluster model, and may be useful to investigate
sharp threshold phenomena for more general dependent
percolation processes, in any dimension.
[37]
Higher order
corrections for anisotropic bootstrap percolation with A.
C. D. van Enter and T. Hulshof,
PTRF
.
×
Higher order corrections for anisotropic bootstrap
percolation.
with A. C. D. van Enter and T. Hulshof,
Probability
Theory and Related Fields, 172(1-2), 191–243, 2018.
 © Tim Hulshof
© Tim Hulshof
Abstract. We study the
critical probability for the metastable phase
transition of the two-dimensional anisotropic
bootstrap percolation model with $(1,2)$-neighbourhood
and threshold $r = 3$. The first order asymptotics for
the critical probability were recently determined by
the first and second authors. Here we determine the
following sharp second and third order asymptotics: \[
p_c\big( [L]^2,\mathcal{N}_{(1,2)},3 \big) \; = \;
\frac{(\log \log L)^2}{12\log L} \, - \, \frac{\log
\log L \, \log \log \log L}{ 3\log L}
+ \frac{\left(\log \frac{9}{2} + 1 \pm o(1)
\right)\log \log L}{6\log L}. \] We note that the
second and third order terms are so large that the
first order asymptotics fail to approximate $p_c$ even
for lattices of size well beyond $10^{10^{1000}}$.
[36]
The box-crossing
property for critical two-dimensional oriented percolation
with V. Tassion and A. Teixeira,
PTRF.
×
The box-crossing property for critical two-dimensional
oriented percolation.
with V. Tassion and A. Teixeira,
Probability
Theory and Related Fields, 171(3–4), 685–708, 2018.
Abstract. We consider critical
oriented Bernoulli percolation on the square lattice
$\mathbb{Z}^2$. We prove a Russo-Seymour-Welsh type result
which allows us to derive several new results concerning
the critical behavior: - We establish that the probability
that the origin is connected to distance $n$ decays
polynomially fast in $n$. - We prove that the critical
cluster of the origin conditioned to survive to distance
$n$ has a typical width $w_n$ satisfying $\epsilon n^{2/5}
< w_n < n^{1-\epsilon}$ for some $\epsilon > 0$.
The sub-linear polynomial fluctuations contrast with the
supercritical regime where $w_n$ is known to behave
linearly in $n$. It is also different from the critical
picture obtained for non-oriented Bernoulli percolation,
in which the scaling limit is non-degenerate in both
directions. All our results extend to the graphical
representation of the one-dimensional contact process.

© Vincent Tassion
[35]
Brochette
percolation with M. Hilario, G. Kozma and V. Sidoravicius,
Israel J. Math.
×
Brochette percolation.
with M. Hilario, G. Kozma and V. Sidoravicius,
Israel
Journal of Mathematics, 225(1), 479–501, 2018.
Abstract. We study bond
percolation on the square lattice with one-dimensional
inhomogeneities. Inhomogeneities are introduced in the
following way: A vertical column on the square lattice is
the set of vertical edges that project to the same vertex
on $\mathbb{Z}$. Select vertical columns at random
independently with a given positive probability. Keep
(respectively remove) vertical edges in the selected
columns, with probability $p$, (respectively $1-p$). All
horizontal edges and vertical edges lying in unselected
columns are kept (respectively removed) with probability
$q$, (respectively $1-q$). We show that, if $p >
p_c(\mathbb{Z}^2)$ (the critical point for homogeneous
Bernoulli bond percolation) then $q$ can be taken strictly
smaller then $p_c(\mathbb{Z}^2)$ in such a way that the
probability that the origin percolates is still positive.
[34]
Sharp phase
transition for the random-cluster and Potts models via
decision trees with A. Raoufi and V. Tassion
Ann.
Math. (2019).
×
Sharp phase transition for the random-cluster and Potts
models via decision trees.
with Aran Raoufi and Vincent Tassion,
Annals
of Mathematics, 189(1), 2019.

Abstract. We prove an
inequality on decision trees on monotonic measures
which generalizes the OSSS inequality on product
spaces. As an application, we use this inequality to
prove a number of new results on lattice spin models
and their random-cluster representations. More
precisely, we prove that
- For the Potts model on transitive graphs,
correlations decay exponentially fast below
criticality.
- For the random-cluster model with cluster weight
$q\ge1$ on transitive graphs, correlations decay
exponentially fast in the subcritical regime and the
cluster-density satisfies the mean-field lower bound
in the supercritical regime.
- For the random-cluster models with cluster weight
$q\ge1$ on planar quasi-transitive graphs $\mathbb G$,
$\frac{p_c(\mathbb G)p_c(\mathbb G^*)}{(1-p_c(\mathbb
G))(1-p_c(\mathbb G^*))}~=~q.$
As a special case, we obtain the value of the critical
point for the square, triangular and hexagonal
lattices (this provides a short proof of the result of
Beffara and Duminil-Copin. These results have many
applications for the understanding of the subcritical
(respectively disordered) phase of all these models.
The techniques developed in this paper have potential
to be extended to a wide class of models including the
Ashkin-Teller model, continuum percolation models such
as Voronoi percolation and Boolean percolation,
super-level sets of massive Gaussian Free Field, and
random-cluster and Potts model with infinite range
interactions.
[33]
Universality for
the random-cluster model on isoradial graphs with J.-H. Li
and I. Manolescu.
EJP (2018).
×
Universality for the random-cluster model on isoradial
graphs.
with Jhih-Huang Li and Ioan Manolescu,
Electronic
Journal of Probability number 23 article 96, 1-70,
2018.
Abstract. We show that the
canonical random-cluster measure associated to
isoradial graphs is critical for all $q \geq 1$.
Additionally, we prove that the phase transition of
the model is of the same type on all isoradial graphs:
continuous for $1 \leq q \leq 4$ and discontinuous for
$q > 4$. For $1 \leq q \leq 4$, the arm exponents
(assuming their existence) are shown to be the same
for all isoradial graphs. In particular, these
properties also hold on the triangular and hexagonal
lattices. Our results also include the limiting case
of quantum random-cluster models in $1+1$ dimensions.

[32]
The sharp
threshold for the Duarte model with B. Bollobás, R. Morris
and P. Smith,
Ann. Prob. (2017).
×
The sharp threshold for the Duarte model.
with B. Bollobás, R. Morris and P. Smith,
Annals
of Probability , 45(6B), 4222-4272, 2017.
Abstract. The class of
critical bootstrap percolation models in two
dimensions was recently introduced by Bollobás, Smith
and Uzzell, and the critical threshold for percolation
was determined up to a constant factor for all such
models by the authors of this paper. Here we develop
and refine the techniques introduced in that paper in
order to determine a sharp threshold for the Duarte
model. This resolves a question of Mountford from
1995, and is the first result of its type for a model
with drift.
[31]
Exponential decay
of loop lengths in the loop $ O (n) $ model with large $ n$
with R. Peled, W. Samotij, Y. Spinka,
CMP
(2017).
×
Exponential decay of loop lengths in the loop $ O (n) $
model with large $ n$.
with R. Peled, W. Samotij, Y. Spinka,
Communications
in Mathematical Physics, 349(3), 777- 817, 2017.
Abstract. The loop $O(n)$
model is a model for a random collection of
non-intersecting loops on the hexagonal lattice, which
is believed to be in the same universality class as
the spin $O(n)$ model. It has been conjectured that
both the spin and the loop $O(n)$ models exhibit
exponential decay of correlations when $n>2$. We
verify this for the loop $O(n)$ model with large
parameter $n$, showing that long loops are
exponentially unlikely to occur, uniformly in the edge
weight $x$. Our proof provides further detail on the
structure of typical configurations in this regime.
Putting appropriate boundary conditions, when $nx^6$
is sufficiently small, the model is in a dilute,
disordered phase in which each vertex is unlikely to
be surrounded by any loops, whereas when $nx^6$ is
sufficiently large, the model is in a dense, ordered
phase which is a small perturbation of one of the
three ground states.
[30]
Continuity of the
phase transition for planar random-cluster and Potts models
with $1\le q\le 4$ with V. Sidoravicius and V. Tassion,
CMP
(2017).
×
Continuity of the phase transition for planar
random-cluster and Potts models with $1\le q\le 4$.
with V. Sidoravicius and V. Tassion,
Communications
in Mathematical Physics, 349(1), 47-107, 2017.

Abstract. This article studies
the planar Potts model and its random-cluster
representation. We show that the phase transition of
the nearest-neighbor ferromagnetic $q$-state Potts
model on $\mathbb Z^2$ is continuous for
$q\in\{2,3,4\}$, in the sense that there exists a
unique Gibbs state, or equivalently that there is no
ordering for the critical Gibbs states with
monochromatic boundary conditions. The proof uses the
random-cluster model with cluster-weight $q\ge1$ (note
that $q$ is not necessarily an integer) and is based
on two ingredients: The fact that two-point function
for the free state decays sub-exponentially fast for
cluster-weights $1\le q\le 4$, which is derived
studying parafermionic observables on a discrete
Riemann surface. And a new result proving the
equivalence of several properties of critical
random-cluster models: - the absence of
infinite-cluster for wired boundary conditions,
- the uniqueness of infinite-volume measures,
- the sub-exponential decay of the two-point function
for free boundary conditions,
- a Russo-Seymour-Welsh type result on crossing
probabilities in rectangles with {\em arbitrary
boundary conditions}.
The result leads to a number of consequences
concerning the scaling limit of the random-cluster
model with $q\in[1,4]$. It shows that the family of
interfaces (for instance for Dobrushin boundary
conditions) are tight when taking the scaling limit
and that any sub-sequential limit can be parametrized
by a Loewner chain. We also study the effect of
boundary conditions on these sub-sequential limits.
Let us mention that the result should be instrumental
in the study of critical exponents as well.
[29]
Conformal
invariance of crossing probabilities for the Ising model with
free boundary conditions with S. Benoist and C. Hongler,
Ann.
IHP (2016).
×
Conformal invariance of crossing probabilities for the
Ising model with free boundary conditions.
with S. Benoist and C. Hongler,
Annales
de l'Institut Henri Poincaré, 52(4), 1784-1798,
2016.
Abstract. We prove that crossing
probabilities for the critical planar Ising model with
free boundary conditions are conformally invariant in the
scaling limit. We do so by establishing the convergence of
certain exploration processes towards
SLE$(3,\frac{-3}2,\frac{-3}2)$. We also derive results on
the exploration tree introduced by Sheffield.

[28]
A new proof of
the sharpness of the phase transition for Bernoulli
percolation on $\mathbb Z^d$ with V. Tassion,
Ens.
Math. (2016).
×
A new proof of the sharpness of the phase transition for
Bernoulli percolation on $\mathbb Z^d$.
with V. Tassion,
L'Enseignement
Mathématique, 62(1/2), 199-206, 2016.
Abstract. We provide a new proof
of the sharpness of the phase transition for
nearest-neighbour Bernoulli percolation. More precisely,
we show that for $p < p_c$, the probability that the
origin is connected by an open path to distance $n$ decays
exponentially fast in $n$, and for $p>p_c$, the
probability that the origin belongs to an infinite cluster
satisfies the mean-field lower bound $\theta(p)\ge
\tfrac{p-p_c}{p(1-p_c)}$. This note presents the argument
of "A new proof of sharpness of the phase transition for
Bernoulli percolation and the Ising model", which is valid
for long-range Bernoulli percolation (and for the Ising
model) on arbitrary transitive graphs in the simpler
framework of nearest-neighbour Bernoulli percolation on
$\mathbb Z^d$.
[27]
A new proof of
the sharpness of the phase transition for Bernoulli
percolation and the Ising model with V. Tassion,
CMP
(2016).
×
A new proof of the sharpness of the phase transition for
Bernoulli percolation and the Ising model.
with V. Tassion,
Communications
in Mathematical Physics, 343(2), 725-745, 2016.
Abstract. We provide a new proof
of the sharpness of the phase transition for Bernoulli
percolation and the Ising model. The proof applies to
infinite range models on arbitrary locally finite
transitive infinite graphs. For Bernoulli percolation, we
prove finiteness of the susceptibility in the subcritical
regime $\beta<\beta_c$, and the mean-field lower bound
$\mathbb P_\beta[0\longleftrightarrow\infty]\ge
(\beta-\beta_c)/\beta$ for $\beta>\beta_c$. For
finite-range models, we also prove that for any
$\beta<\beta_c$, the probability of an open path from
the origin to distance $n$ decays exponentially fast in
$n$. For the Ising model, we prove finiteness of the
susceptibility for $\beta<\beta_c$, and the mean-field
lower bound $\langle \sigma_0\rangle_\beta^+\ge
\sqrt{(\beta^2-\beta_c^2)/\beta^2}$ for
$\beta>\beta_c$. For finite-range models, we also prove
that the two-point correlations functions decay
exponentially fast in the distance for $\beta<\beta_c$.
[26]
On the
probability that self-avoiding walk ends at a given point
with A. Glazman, A. Hammond, and I. Manolescu
Ann.
Prob. (2016).
×
On the probability that self-avoiding walk ends at a
given point.
with A. Glazman, A. Hammond, and I. Manolescu,
Annals
of probability, 44(2), 955-983, 2016.
Abstract. We prove two results on
the delocalization of the endpoint of a uniform
self-avoiding walk on $\mathbb Z^d$ for $d\ge2$. We show
that the probability that a walk of length n ends at a
point $x$ tends to $0$ as $n$ tends to infinity, uniformly
in $x$. Also, when $x$ is fixed, with $\|x\|=1$, this
probability decreases faster than $n^{-1/4+\varepsilon}$
for any $\varepsilon>0$. This provides a bound on the
probability that a self-avoiding walk is a polygon.
[25]
A quantitative
Burton-Keane estimate under strong FKG condition with D.
Ioffe and Y. Velenik,
Ann.
Prob. (2016).
×
A quantitative Burton-Keane estimate under strong FKG
condition.
with D. Ioffe and Y. Velenik,
Annals of Probability, 44(5), 3335-3356, 2016.
Abstract. We consider
translationally-invariant percolation models on
$\mathbb Z^d$ satisfying the finite energy and the FKG
properties. We provide explicit upper bounds on the
probability of having two distinct clusters going from
the endpoints of an edge to distance $n$ (this
corresponds to a finite size version of the celebrated
Burton-Keane argument proving uniqueness of the
infinite-cluster). The proof is based on the
generalization of a reverse Poincaré inequality proved
by Sen and Chatterjee. As a consequence, we obtain
upper bounds on the probability of the so-called
four-arm event for planar random-cluster models with
cluster-weight $q\geq1$.
[24]
The phase
transitions of the planar random-cluster and Potts models with
$q\ge1$ are sharp with I.Manolescu,
PTRF
(2016).
×
The phase transitions of the planar random-cluster and
Potts models with $q\ge1$ are sharp.
with I.Manolescu,
Probability
Theory and Related Fields, 164(3), 865-892, 2016.
Abstract. We prove that
random-cluster models with $q \geq 1$ on a variety of
planar lattices have a sharp phase transition, that is
that there exists some parameter $p_c$ below which the
model exhibits exponential decay and above which there
exists a.s. an infinite cluster. The result may be
extended to the Potts model via the Edwards-Sokal
coupling. Our method is based on sharp threshold
techniques and certain symmetries of the lattice; in
particular it makes no use of self-duality. Due to its
nature, this strategy could be useful in studying
other planar models satisfying the FKG lattice
condition and some additional differential
inequalities.
[23]
Crossing
probabilities in topological rectangles for the critical
planar FK-Ising model with D. Chelkak and C. Hongler,
EJP
(2016).
×
Crossing probabilities in topological rectangles for the
critical planar FK-Ising model.
with D. Chelkak and C. Hongler,
Electronic
Journal of Probability, 21(1), 1-28, 2016.
Abstract. We consider the FK-Ising
model in two dimensions at criticality. We obtain RSW-type
crossing probabilities bounds in arbitrary topological
rectangles, uniform with respect to the boundary
conditions, generalizing existing results. Our result
relies on new discrete complex analysis techniques,
introduced in a previous paper by Chelkak. We detail some
applications, in particular the computation of so-called
universal exponents and crossing bounds for the classical
Ising model.
[22]
Absence of
infinite cluster for critical Bernoulli percolation on slabs
with V. Sidoravicius and V. Tassion,
CPAM
(2016).
×
Absence of infinite cluster for critical Bernoulli
percolation on slabs.
with V. Sidoravicius and V. Tassion,
Communications
in Pure and Applied Mathematics, 69(7), 1397-1411,
2016.
Abstract. We prove that for
Bernoulli percolation on a graph $\mathbb
Z^2\times\{0,\dots,k\}$, $k\ge0$, there is no infinite
cluster at criticality, almost surely. The proof extends
to finite range Bernoulli percolation models on $\mathbb
Z^2$ which are invariant under $\pi/2$-rotation and
reflection.
[21]
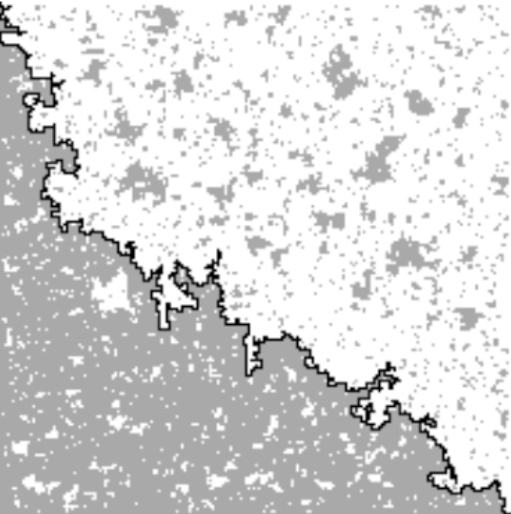
Disorder, entropy
and harmonic functions with I. Benjamini, G. Kozma and A.
Yadin,
Ann. Prob.
(2015).
×
Disorder, entropy and harmonic functions.
with I. Benjamini, G. Kozma and A. Yadin,
Annals
of Probability, 43(5), 2332-2373, 2015.
Abstract. We study harmonic
functions on random environments with particular
emphasis on the case of the infinite cluster of
supercritical percolation on $\mathbb Z^d$. We prove
that the vector space of harmonic functions growing at
most linearly is $d+1$-dimensional almost surely. In
particular, there are no non-constant sublinear
harmonic functions (thus implying the uniqueness of
the corrector). The main ingredient of the proof is
given by a quantitative, annealed version of the
Kaimanovich-Vershik entropy argument. This also
provides bounds on the derivative of the heat kernel,
simplifying and generalizing existing results. Even
reversibility is not necessary. We also mention
several open problems and conjectures on the behavior
of harmonic functions on stationary random graphs.
[20]
Random currents
and continuity of Ising model's spontaneous magnetization
with M. Aizenman and V. Sidoravicius,
CMP
(2015).
×
Random currents and continuity of Ising model's
spontaneous magnetization.
with M. Aizenman and V. Sidoravicius,
Communications
in Mathematical Physics, 334, 719-742, 2015.
Abstract. The spontaneous
magnetization is proved to vanish continuously at the
critical temperature for a class of ferromagnetic
Ising spin systems which includes the nearest neighbor
ferromagnetic Ising spin model on $\mathbb Z^d$ in
$d=3$ dimensions. The analysis applies also to higher
dimensions, for which the result is already known, and
to systems with interactions of power law decay. The
proof employs in an essential way an extension of
Ising model's random current representation to
the model's infinite volume limit. Using it, we relate
the continuity of the magnetization to the vanishing
of the free boundary condition Gibbs state's Long
Range Order parameter. For reflection positive models
the resulting criterion for continuity may be
established through the infrared bound for all but the
borderline case, of the one dimensional model with
$1/r^2$ interaction, for which the spontaneous
magnetization is known to be discontinuous at $T_c$.
[19]
On the critical
parameters of the $q\ge4$ random-cluster model on isoradial
graphs with V. Beffara and S. Smirnov,
J. Phys. A (2015).
×
On the critical parameters of the $q\ge4$ random-cluster
model on isoradial graphs.
with V. Beffara and S. Smirnov,
Journal Physics A: Mathematical and Theoretical,
48(48), 484003, 2015.
Abstract. The critical surface
for random-cluster model with cluster-weight $q\ge 4$
on isoradial graphs is identified using parafermionic
observables. Correlations are also shown to decay
exponentially fast in the subcritical regime. While
this result is restricted to random-cluster models
with $q\ge 4$, it extends the recent theorem of [BD12]
to a large class of planar graphs. In particular, the
anisotropic random-cluster model on the square lattice
are shown to be critical if
$\frac{p_vp_h}{(1-p_v)(1-p_h)}=q$, where $p_v$ and
$p_h$ denote the horizontal and vertical edge-weights
respectively. We also provide consequences for Potts
models.
Comment. This article is part of the special
issue Exactly solved models and beyond in
honour of R. Baxter's 75th birthday.
[18]
Convergence of
Ising interfaces to Schramm's SLE curves with D. Chelkak,
C. Hongler, A. Kemppainen and S. Smirnov,
Comptes
Rendus Math. (2014).
×
Convergence of Ising interfaces to Schramm's SLE curves.
with D. Chelkak, C. Hongler, A. Kemppainen and S. Smirnov,
Comptes
Rendus Mathematique, 352(2), 157-161, 2014.
Abstract. We show how to combine
our earlier results to deduce strong convergence of the
interfaces in the planar critical Ising model and its
random-cluster representation to Schramm’s SLE curves with
parameter $\kappa=3$ and $\kappa=16/3$ respectively.
[17]
The critical
fugacity for surface adsorption of self-avoiding walks on the
honeycomb lattice is $1+\sqrt 2$ with N. Beaton, M.
Bousquet-Mélou, J. De Gier and A. Guttmann,
CMP
(2014).
×
The critical fugacity for surface adsorption of
self-avoiding walks on the honeycomb lattice is $1+\sqrt
2$.
with N. Beaton, M. Bousquet-Mélou, J. De Gier and A.
Guttmann,
Communications
in Mathematical Physics, 326(3), 727-754, 2014.
(2018 Gavin Brown Prize of the Australian Mathematical
Society.)
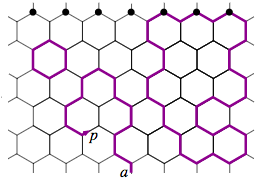
Abstract. In 2010, the growth
constant of self-avoiding walks on the hexagonal
(a.k.a honeycomb) lattice was proved to be equal to�
$\sqrt{2+\sqrt 2}$: A key identity used in that proof
was later generalised by Smirnov so as to apply to a
general $O(n)$ loop model with $n\in[-2,2]$ (the case
$n = 0$ corresponding to self-avoiding walks). We
modify this model by restricting to a half-plane and
introducing a fugacity associated with boundary sites
(also called surface sites) and obtain a
generalisation of Smirnov’s identity. The value of the
critical surface fugacity was conjectured by Batchelor
and Yung in 1995. This value also plays a crucial role
in our identity, which thus provides an independent
prediction for it. For the case $n = 0$, corresponding
to self-avoiding walks interacting with a surface, we
prove the conjectured value of the critical surface
fugacity. A critical part of this proof involves
demonstrating that the generating function of
self-avoiding bridges of height $T$, taken at its
critical point�, tends to 0 as $T$ increases, as
predicted from SLE theory.
[16]
Seven-dimensional
forest fires with D. Ahlberg, G. Kozma and V.
Sidoravicius,
Ann. IHP
(2014).
×
Seven-dimensional forest fires.
with D. Ahlberg, G. Kozma and V. Sidoravicius,
Annales
de l'Institut Henri Poincaré, 50(2), 315-326, 2014.
Abstract. We show that in high
dimensional Bernoulli percolation, removing from a thin
infinite cluster a much thinner infinite cluster leaves an
infinite component. This observation has implications for
the van den Berg-Brouwer forest fire process, also known
as self-destructive percolation, for dimension high
enough.
[15]
The near-critical
planar FK-Ising model with C. Garban and G. Pete,
CMP
(2014).
×
The near-critical planar FK-Ising model.
with C. Garban and G. Pete,
Communications
in Mathematical Physics, 326, 1-35, 2014.
Abstract. We study the
near-critical FK-Ising model. First, a determination of
the correlation length defined via crossing probabilities
is provided. Second, a phenomenon about the near-critical
behavior of FK-Ising is highlighted, which is completely
missing from the case of standard percolation: in any
monotone coupling of FK configurations (e.g., in the one
introduced by Grimmett), as one raises $p$ near $p_c$, the
new edges arrive in a self-organized way, so that the
correlation length is not governed anymore by the number
of pivotal edges at criticality.
[14]
Supercritical
self-avoiding walks are space-filling with G. Kozma and A.
Yadin,
Ann. IHP
(2014).
×
Supercritical self-avoiding walks are space-filling.
with G. Kozma and A. Yadin,
Annales
de l'Institut Henri Poincaré, 50(2), 315-326, 2014.
Abstract. We consider random
self-avoiding walks between two points on the boundary of
a finite subdomain of $\mathbb Z^d$ (the probability of a
self-avoiding trajectory gamma is proportional to
$\mu^{-\ell(\gamma)}$). We show that the random trajectory
becomes space-filling in the scaling limit when the
parameter mu is supercritical.
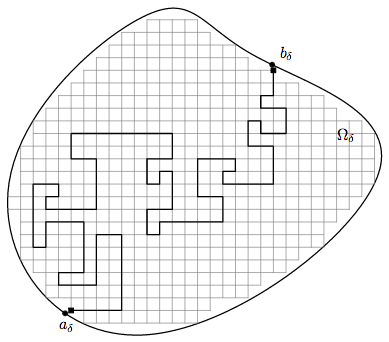
[13]
On the Gibbs
states of the noncritical Potts model on $\mathbb Z^2$
with L. Coquille, D. Ioffe and Y. Velenik,
PTRF
(2014).
×
On the Gibbs states of the noncritical Potts model on
$\mathbb Z^2$.
with L. Coquille, D. Ioffe and Y. Velenik,
Probability
Theory and Related Fields, 158(1-2), 477-512, 2014.
Abstract. We prove that all
Gibbs states of the q-state nearest neighbor Potts
model on $\mathbb Z^2$ below the critical temperature
are convex combinations of the $q$ pure phases; in
particular, they are all translation invariant. To
achieve this goal, we consider such models in large
finite boxes with arbitrary boundary condition, and
prove that the center of the box lies deeply inside a
pure phase with high probability. Our estimate of the
finite-volume error term is of essentially optimal
order, which stems from the Brownian scaling of
fluctuating interfaces. The results hold at any
supercritical value of the inverse temperature $\beta
\ge\beta_c$.
© Vincent Beffara
[12]
Self-avoiding
walk is sub-ballistic with A. Hammond,
CMP
(2013).
×
Self-avoiding walk is sub-ballistic.
with A. Hammond,
Communications
in Mathematical Physics, 324(2), 401-423, 2013.
Abstract. We prove that
self-avoiding walk on $\mathbb Z^d$ is sub-ballistic in
any dimension $d\ge2$.
[11]
The critical
temperature for the Ising model on planar doubly periodic
graphs with D. Cimasoni,
EJP
(2013).
×
The critical temperature for the Ising model on planar
doubly periodic graphs.
with D. Cimasoni,
Electronic
Journal in Probability, 18(44), 1-18, 2013.
Abstract. We provide a simple
characterization of the critical temperature for the Ising
model on an arbitrary planar doubly periodic weighted
graph. More precisely, the critical inverse temperature
$\beta$ for a graph $G$ with coupling constants
$(J_e)_{e\in E(G)}$ is obtained as the unique solution of
a linear equation in the variables $(\tanh(\beta
J_e))_{e\in E(G)}$. This is achieved by studying the
high-temperature expansion of the model using Kac-Ward
matrices.

[10]
Limit of the
Wulff Crystal when approaching criticality for site
percolation on the triangular lattice ECP
(2013).
×
Limit of the Wulff Crystal when approaching criticality
for site percolation on the triangular lattice.
Electronic Communications
in Probability, 18(93), 1–9, 2013.
Abstract. When a model is
conformally invariant (hence rotationally invariant) at
criticality, theWulff crystal is expected to become
isotropic as the parameter tends to the critical one.
Nevertheless, known proofs of conformal invariance give
very little information on the isotropy of off critical
regimes. The aim of this small note is to derive the
convergence of the Wulff crystal to a disk for site
percolation on the triangular lattice. The main ingredient
of the proof is the result of Garban, Pete and Schramm
[GPS] on the rotational invariance of the near-critical
scaling limit.
[9]
Containing
Internal Diffusion Limited Aggregation with C. Lucas, A.
Yadin and A. Yehudayoff,
ECP
(2013).
×
Containing Internal Diffusion Limited Aggregation.
with C. Lucas, A. Yadin and A. Yehudayoff,
Electronic
Communications in Probability, 18(50), 1-8, 2013.
Abstract. Internal Di�ffusion
Limited Aggregation (IDLA) is a model that describes
the growth of a random aggregate of particles from the
inside out. Shellef proved that IDLA processes on
supercritical percolation clusters of integer-lattices
��fill Euclidean balls, with high probability. In this
article, we complete the picture and prove a
limit-shape theorem for IDLA on such percolation
clusters, by providing the corresponding upper bound.
The technique to prove upper bounds is new and robust:
it only requires the existence of a good lower bound.
Specifi�cally, this way of proving upper bounds on
IDLA clusters is more suitable for random environments
than previous ways, since it does not harness harmonic
measure estimates.
© Cyrille Lucas
[8]
Sharp
metastability threshold for an anisotropic bootstrap
percolation model with A. C. D. van Enter,
Ann.
Prob. (2013).
×
Sharp metastability threshold for an anisotropic
bootstrap percolation model.
with A. C. D. van Enter,
Annals
of Probability, 41(3A), 1218-1242, 2013.

Abstract. Bootstrap
percolation models have been extensively studied
during the two past decades. In this article, we study
an anisotropic bootstrap percolation model. We prove
that it exhibits a sharp metastability threshold. This
is the first mathematical proof of a sharp threshold
for an anisotropic bootstrap percolation model.
Erratum. Although Theorem 1.1 as stated in our
paper is correct, the generalisation announced at the
end of page 1219 and the beginning of page 1220 to the
situation where the site $(m,n)$ gets occupied if
$k+1$ sites among the $2k+2$ sites $(m+1,n)$,
$(m,n-1)$ and $(m-k,n),\dots, (m-1,n),
(m+1,n),\dots,(m+k,n)$ are occupied, is incorrect as
stated for $k\ge2$. It should read that (in
probability) $\frac{1}{p}(\log \frac{1}{p})^2 \log T$
tends to $\frac{(k-1)^2}{4(k+1)}$. In other words, the
constant $\frac{1}{4(k+1)}$ given in our paper on the
right-hand side of the unnumbered equation on page
1220 should be replaced by $\frac{(k-1)^2}{4(k+1)}$.
We thank Rob Morris for pointing this out to us.
Also, the definition of weakly connected in page 1226
should be modified as follows. Two occupied points
$x,y\in\mathbb Z^2$ are weakly connected if $x\in
y+\mathcal N$ or there exists $z\in \mathbb Z^2$ such
that $x,y\in z+\mathcal N$. We thank Tim Hulshof for
pointing this out to us.
[7]
The connective
constant of the honeycomb lattice equals $\sqrt{2+\sqrt 2}$
with S. Smirnov,
Ann. Math.
(2012).
×
The connective constant of the honeycomb lattice equals
$\sqrt{2+\sqrt 2}$.
with S. Smirnov,
Annals
of Mathematics (2), 175(3), 1653-1665, 2012.
Abstract. We provide the
fi�rst mathematical proof that the connective constant
of the hexagonal lattice is equal to $\sqrt{2+\sqrt
2}$. This value has been derived non rigorously by B.
Nienhuis in 1982, using Coulomb gas approach from
theoretical physics. Our proof uses a parafermionic
observable for the self avoiding walk, which
satisfi�es a half of the discrete Cauchy-Riemann
relations. Establishing the other half of the
relations (which conjecturally holds in the scaling
limit) would also imply convergence of the
self-avoiding walk to SLE(8/3).
© Vincent Beffara
[6]
Divergence of the
correlation length for critical planar FK percolation with
$1\le q\le 4$ via parafermionic observables J.
Phys. A (2012).
×
Divergence of the correlation length for critical planar
FK percolation with $1\le q\le 4$ via parafermionic
observables.
Journal Physics A:
Mathematical and Theoretical, 45 494013, 26 pages,
2012.
Abstract. Parafermionic
observables were introduced by Smirnov for planar FK
percolation in order to study the critical phase $(p;
q) = (p_c(q); q)$. This article gathers several known
properties of these observables. Some of these
properties are used to prove the divergence of the
correlation length when approaching the critical point
for FK percolation when $1\le q\le 4$. A crucial step
is to consider FK percolation on the universal cover
of the punctured plane. We also mention several
conjectures on FK percolation with arbitrary
cluster-weight $q \ge 0$.
Comment. This article is part of 'Lattice
models and integrability', a special issue of Journal
of Physics A: Mathematical and Theoretical in honour
of F Y Wu's 80th birthday.
[5]
The self-dual
point of the two-dimensional random-cluster model is critical
for $q\ge1$ with V. Beffara,
PTRF
(2012).
×
The self-dual point of the two-dimensional random-cluster
model is critical for $q\ge1$.
with V. Beffara,
Probability
Theory and Related Fields, 153(3), 511-542, 2012.
Abstract. We prove a
long-standing conjecture on random-cluster models,
namely that the critical point for such models with
parameter $q\ge1$ on the square lattice is equal to
the self-dual point $p_{\rm
sd}(q)=\sqrt{q}/(1+\sqrt{q})$. This gives a rigorous
proof that the critical temperature of the q-state
Potts model is equal to $\log(1+\sqrt q)$ for all
$q\ge 1$. We further prove that the transition is
sharp, meaning that there is exponential decay of
correlations in the sub-critical phase. The techniques
of this paper are rigorous and valid for all $q\ge1$,
in contrast to earlier methods valid only for certain
given $q$. The proof extends to the triangular and the
hexagonal lattices as well.
[4]
The sharp
threshold for bootstrap percolation in all dimensions with
J. Balogh, B. Bollobás, and R. Morris,
Trans.
AMS (2012).
×
The sharp threshold for bootstrap percolation in all
dimensions.
with J. Balogh, B. Bollobás, and R. Morris,
Transaction
of the American Mathematical Society, 364,
2667-2701, 2012.
Abstract. In $r$-neighbour
bootstrap percolation on a graph $G$, a (typically
random) set $A$ of initially 'infected' vertices
spreads by infecting (at each time step) vertices with
at least $r$ already-infected neighbours. This process
may be viewed as a monotone version of the Glauber
dynamics of the Ising model, and has been extensively
studied on the $d$-dimensional grid $[n]^d$. The
elements of the set $A$ are usually chosen
independently, with some density $p$, and the main
question is to determine $p_c([n]^d,r)$, the density
at which percolation (infection of the entire vertex
set) becomes likely.
In this paper we prove, for every pair $d \ge r \ge
2$, that there is a constant $L(d,r)$ such that
$p_c([n]^d,r) = [(L(d,r) + o(1)) / log_(r-1)
(n)]^{d-r+1}$ as $n \to \infty$, where $log_r$ denotes
an $r$-times iterated logarithm. We thus prove the
existence of a sharp threshold for percolation in any
(fixed) number of dimensions. Moreover, we determine
$L(d,r)$ for every pair $(d,r)$.
[3]
Smirnov's
fermionic observable away from criticality with V.
Beffara,
Ann. Prob.
(2012).
×
Smirnov's fermionic observable away from criticality.
with V. Beffara,
Annals
of Probability, 40(6), 2667-2689, 2012.
Abstract. In a recent and
celebrated article, Smirnov defines an observable for the
self-dual random-cluster model with cluster weight $q = 2$
on the square lattice $\mathbb Z^2$ , and uses it to
obtain conformal invariance in the scaling limit. We study
this observable away from the self-dual point. From this,
we obtain a new derivation of the fact that the self-dual
and critical points coincide, which implies that the
critical inverse temperature of the Ising model equals
$\tfrac12 \log(1 + \sqrt 2)$. Moreover, we relate the
correlation length of the model to the large deviation
behavior of a certain massive random walk (thus confirming
an observation by Messikh), which allows us to compute it
explicitly.
[2]
Connection
probabilities and RSW-type bounds for the two-dimensional FK
Ising model with C. Hongler, P. Nolin,
CPAM
(2011).
×
Connection probabilities and RSW-type bounds for the
two-dimensional FK Ising model.
with C. Hongler, P. Nolin,
Communications
in Pure and Applied Mathematics, 64(9), 1165-1198,
2011.
Abstract. We prove
Russo-Seymour-Welsh-type uniform bounds on crossing
probabilities for the FK Ising (FK percolation with
cluster weight $q = 2$) model at criticality, independent
of the boundary conditions. Our proof relies mainly on
Smirnov’s fermionic observable for the FK Ising model,
which allows us to get precise estimates on boundary
connection probabilities. We stay in a discrete setting,
in particular we do not make use of any continuum limit,
and our result can be used to derive directly several
noteworthy properties – including some new ones – among
which the fact that there is no infinite cluster at
criticality, tightness properties for the interfaces, and
the existence of several critical exponents, in particular
the half-plane one-arm exponent. Such crossing bounds are
also instrumental for important applications such as
constructing the scaling limit of the Ising spin field, and
deriving polynomial bounds for the mixing time of the
Glauber dynamics at criticality.
Comment. This version differs slightly from the
published version. A mistake is fixed in the proof of
Proposition 5.11. We thank C. Garban for bringing the
mistake to our attention and for useful discussions.
[1]
Bridge
decomposition of Restriction Measures with T. Alberts,
J.
Stat. Phys. (2010).
×
Bridge decomposition of Restriction Measures
with T. Alberts,
Journal
of Statistical Physics, 140, 467-493, 2010.
Abstract. Motivated by
Kesten’s bridge decomposition for two-dimensional
self-avoiding walks in the upper half plane, we show
that the conjectured scaling limit of the half-plane
SAW, the SLE(8/3) process, also has an appropriately
defined bridge decomposition. This continuum
decomposition turns out to entirely be a consequence
of the restriction property of SLE(8/3), and as a
result can be generalized to the wider class of
restriction measures. Specifically we show that the
restriction hulls with index less than one can be
decomposed into a Poisson Point Process of irreducible
bridges in a way that is similar to Ito’s excursion
decomposition of a Brownian motion according to its
excursions.
© Tom Alberts